Articles
Selecting materials for H2 service
Hydrogen Equipment and Services
M. ORTOLANI and P. BORTOT, Tenaris
Hydrogen (H2) is widely regarded as a key ingredient in the transition to carbon-free energy. Besides not releasing greenhouse gases (GHGs) upon combustion, it possesses an elevated energy density per unit mass of 120 MJ/kg,1 which is far superior to common fossil fuels such as methane (55.7 MJ/kg)2 or coal (~14 MJ/kg–36 MJ/kg).3 Conversely, H2 has an extremely low density: at 1 atm (atmosphere) pressure and 20°C, the mass density of H2 is only 0.0827 kg/m3 vs. 0.659 kg/m3 for methane;4,5 or, in terms of volumetric energy density, 9.9 MJ/m3 for H2 vs. 36.7 MJ/m3 for methane.
Consequently, H2 must be stored at more elevated pressures with respect to hydrocarbon gas fuels to achieve similar energy density. This creates higher requirements on pressure vessels and containment structures, both in terms of building materials’ strength and assembly weight. Moreover, H2 is known to degrade materials’ properties; in particular, in the case of metals, molecular H2 can dissociate at the metal surface, resulting in atomic H2 to diffuse into the bulk. This can eventually degrade the material’s properties, especially the ductility and fracture resistance. This phenomenon is called H2 embrittlement (HE)6,7,8 and is especially relevant for the most popular and readily available class of construction materials (i.e., carbon and alloy ferritic steels).
Intuitively, HE is more or less severe depending on the actual environment, defined in terms of composition (i.e., pure H2 or blend, presence and type of contaminants), temperature and pressure. Moreover, HE is more or less critical depending on the loading conditions (i.e., constant loading or cyclic service, uniaxial or multiaxial loads, stress concentration). Finally, materials show different sensitivity to HE, and similar materials from the same class can result in quite different behavior: differences in microstructure, mechanical properties (hardness) and homogeneity may determine a material’s suitability for service in H2. HE is specific to a combination of environment, loading and material; therefore, an accurate knowledge of materials’ behavior when exposed to H2 is essential to reliable design and operation.
Design and normatives. Since H2 mostly affects a material’s fracture resistance, the design of components should employ fracture mechanics principles and make use of actual material test data to properly quantify safety margins against H2-assisted crack propagation.6 Knowledge of a material’s fracture resistance in a given H2 environment is a prerequisite for the designer. Fracture toughness (FT) can be measured in terms of threshold stress intensity for crack initiation in plane strain conditions, usually indicated as (or in H2); the latter may be calculated from the strain energy release rate (J value) for fracture initiation under plane strain conditions, in which case the nomenclature (or in H2) is used. The measurement is performed under increasing load (or displacement), and the testing procedure is described in detail in ASTM E18209 or ISO 1213510.
Alternatively, FT can be measured in terms of resistance to crack propagation under static loading (KIAPP): the measurement is performed by exposing a pre-cracked specimen—at either a fixed load or a fixed displacement—to the desired environment (H2) and for a specified amount of time. If the material is prone to environment-assisted cracking, the constant load test may fail before the end test duration; in this case, the fracture toughness KTH is defined as the highest KIAPP value that results in no failure after the prescribed duration. In the constant displacement test, the pre-crack may extend until arrest (at the final stress intensity value of KIA < KIAPP), in which case one may define the threshold stress intensity KTH as the minimum KIAPP that results in crack propagation, and the corresponding stress intensity at crack arrest as KTHa. Standardized methods for determination are detailed in ASTM E168111 and ASME BPVC Sec. VIII Div. 3 Article KD-1012. Typically, KTH>KTHa>KJH.13
The material’s FT is used to define the critical crack size, and the corresponding critical stress concentration in H2 K*IH. For example, in the case of the ASME BPVC Sec. VIII, Div. 3, Article KD-10, the critical crack size is determined by (a set of) static loading tests in accordance with ASTM E1681.
The structural integrity of components exposed to H2 is finally assessed by using a failure assessment diagram (FAD) approach14 that is a plot of the loading conditions described in dimensionless terms stress intensity ratio and load ratio Kr = KI ⁄ K*IH and Lr = σ ⁄ σy, and compared against a known loading limit curve.
Cyclic operation must also consider the fatigue crack growth phenomenon, which is assisted by the environment. Hence, fatigue crack growth rates (FCGRs) must be determined for not only a given material and environment combination, but also loading conditions. In fact, the FCGR generally follows the form da/dN = C ΔKm, where da/dN is the crack growth rate; ΔK = Kmax - Kmin is the difference between the maximum and minimum applied stress intensity; and C and m are material-specific coefficients, valid for a given stress intensity ratio R = Kmax ⁄ Kmin, ΔK range, and (most importantly) a specific environment. Testing is regulated by ASTM E64712 or ISO 1210815 standards.
Normative overview: The ASME Boiler and Pressure Vessels Code. Construction of pressure vessels in accordance with the ASME Boiler and Pressure Vessels Code is regulated in Section VIII12, which is articulated in three different divisions detailed here.
Division 1 describes a design-by-rule approach that is more conservative in terms of allowable design stresses and is not recommended (although not prohibited) for vessels operating at pressures > 3,000 psi (> 20 MPa). Division 1 does not provide rules for fatigue analysis (except for bellows expansion joints) nor for service in environment, leaving the designer with the responsibility to account for these service conditions.
Division 2 provides alternative rules for the design and is recommended for vessels operating at up to 10,000 psi (70 MPa); like Division 1, the use of Division 2 is not prohibited for higher pressures. Division 2 provides separate sets of prescriptions for design-by-rule or design-by-analysis, depending on the complexity of the component’s geometry. In Division 2, a fatigue analysis is mandatory, but only following a stress-life (S-N) approach that does not involve fracture mechanics. No rule is provided for the effect of environment, again leaving the responsibility to the designer.
Division 3 provides alternate rules for the design of high-pressure components and is intended for designing vessels operating at pressures of > 10,000 psi (> 70 MPa). Only design-by-analysis is contemplated. Fatigue analysis is mandatory, either using the stress-life (S-N) model but prescribing the fracture mechanics approach where applicable. Specific rules are given for the design of pressure vessels operating with H2 in Article KD-10: the article prescribes material testing in H2 at pressures greater than or equal to the service conditions, including FT measurement in accordance with ASTM E1681 on each material and for each welding procedure, FCGR measurement in accordance with ASTM E647 on each grade of material, and for each welding procedure.
Neither FT nor FCGR data are provided in Section VIII; however, Code Case 2938-1 provides reference values for common pressure vessel construction materials (SA-372 and SA-723 grades). It is worth noting that CC 2938-1 data can be used for H2 up to 15,000 psi (103 MPa), but no parametrization with respect to pressure is given, although the effect of pressure (fugacity) is recognized16—in other words, FT and FCGR are necessarily conservative.
NORMATIVE: EN 13445—UNFIRED PRESSURE VESSELS
The European standard EN 13445 provides rules for unfired pressure vessels; Part 3 specifically deals with design rules. EN 13445-3 is very similar to ASME BPVC Sec. VIII Div. 2: it provides instructions for design-by-rule or design-by-analysis; prescribes fatigue analysis based on the stress-life approach where applicable, without contemplating fracture mechanics assessment; and leaves the designer with the responsibility to consider environmental effects. In other words, no specific indication is given for the design of H2-containing pressure vessels.
Materials. It has already been mentioned that material performance in the presence of H2 gas varies along with the operating environment conditions, but also among similar materials. In the following sections, an overview of the fracture resistance in H2 of several ferritic steels and for different operating conditions is presented.
Factors affecting fracture toughness. It is seen in literature17 that the H2 concentration in the material is proportional to the square root of the environment H2 pressure, or, more accurately, fugacity (Sievert’s law). Therefore, a decrease in material fracture resistance as gas pressure increases can be expected, steeper at low pressures and more level at higher pressures: this is in fact the case, as seen in FIG. 1.
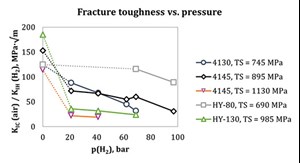
It is also evident how different alloys and grades result in significantly different FT at various pressure levels. It is known that the fracture resistance of ferritic steels in H2 is primarily inversely proportional to tensile strength18; nonetheless, as shown by Ronevich, et al., the fracture resistance of materials with a tensile strength lower than ~900 MPa is quite scattered. Moreover, steels with even lower strength do not necessarily follow the same trend of FT vs. tensile strength (FIG. 2).
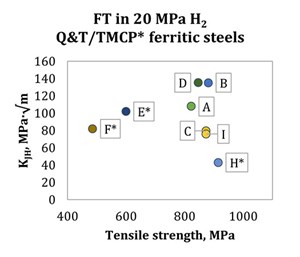
However, the referenced work by Ronevich, et al.,18 demonstrates how, for a single chosen alloy, fracture resistance is a relatively straightforward function of microstructure and strength. The concern about the fracture properties of weldment heat-affected zones (HAZ), therefore, follows naturally; in fact, Article KD-10 of ASME BPVC VIII-3 explicitly requests the testing of the HAZ for each welding procedure used in pressure vessel construction. However, an HAZ consists of a gradient of microstructures arising naturally from the different thermal history as a function of the distance from the fusion line. Care must be taken—in the interest of safe design—to test the portion of the HAZ that is expected to show the worst performance in the service environment: in this case, the highest hardness spot, or the coarse-grained HAZ (CGHAZ).
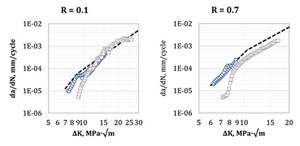
This can be done by manufacturing a test joint with a straight bevel, akin to the API RP-2Z procedure,19 and machine testing specimens with the notch parallel to the fusion line and (as much as possible) within the extent of the CGHAZ. Such a procedure can highlight weak links in a weldment otherwise apparently suitable for service in H2 (see FIG. 3). Note: Fracture properties in air, even if tested at low temperatures, do not correlate to the poor performance of the CGHAZ in H2.

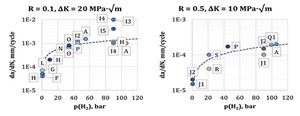
Factors affecting fatigue crack growth rates. Similar to fracture toughness, gas fugacity can be expected to affect a material’s resistance to fatigue crack propagation. This is indeed the case, although it has been observed that the magnitude of this effect differs between low- and high-ΔK range (FIG. 4).23 In fact, it appears that increasing H2 pressure accelerates the transition from a low crack growth rate curve (equivalent to the material’s behavior in air or inert gas) to a high crack growth rate curve specific to the environment’s characteristics.24,25 Again, similar to fracture toughness, FCGR properties of weldments may differ by a significant amount from the corresponding parent material.26
For a given ΔK range, it is eventually possible to calculate a mean relation between H2 gas pressure and FCGR. While it is possible to identify a general, average trend applicable to all ferritic materials, it is apparent how different materials show variable behavior. FIG. 5 shows a compilation of FCGR from different sources.
Takeaways. The main observation presented can be summarized as:
- Materials suffer from mechanical properties de-rating when exposed to H2; in particular, their fracture and fatigue resistance are variably reduced, depending on the environment, the loading conditions and the material’s microstructure.
- Performing a structural integrity assessment for H2 service should involve fracture mechanics evaluations, and requires knowledge of the construction materials’ fracture properties in the service environment.
- Common design standards for pressure equipment (ASME BPVC Sec. VIII Div. 1 and 2; ASME B31.3; EN 13445) leave the responsibility of considering the effect of the operating environment to the designer (FIG. 6). However, other standards contain specific instructions for designing equipment that operates with gaseous H2 (ASME BPVC Sec. VIII Div. 3; ASME B31.12).
- Existing standards that specifically address service with H2 gas also contain some material properties in H2. Such data is often general and non-conservative and does not consider all material (microstructure and hardness) nor environmental (gas pressure) factors.
- Material testing in H2 remains necessary for a better understanding of materials’ behavior, and to eventually select the optimal solution for the construction and operation of pressure equipment.H2T

NOTES
a The Tenaris THera™ technology storage system
LITERATURE CITED
1 Zittel, W., R. Wurster and L. Bolkow, Advantages and Disadvantages of H2. H2 in the Energy Sector, Systemtechnik GmbH, 1996.
2 Dorin, H., P. Demmin and D. L. Gabriel, Chemistry: The Study of Matter, 4th Ed., Prentice Hall Inc., Needham, Massachusetts, 1987.
3 Cooper, B. R. and W. A. Ellingson, The Science and Technology of Coal and Coal Utilization, Springer, Boston, Massachusetts, 1984.
4 The Engineering ToolBox, “Hydrogen—Density and specific weight vs. temperature and pressure,” 2018, online: https://www.engineeringtoolbox.com/H2-H2-density-specific-weight-temperature-pressure-d_2044.html
5 The Engineering ToolBox, “Methane—Density and specific weight vs. temperature and pressure,” 2018, online: https://www.engineeringtoolbox.com/methane-density-specific-weight-temperature-pressure-d_2020.html
6 San Marchi, C. and B. P. Somerday, “Technical reference for hydrogen compatibility of materials,” SAND2012-7321, Sandia National Laboratories, Albuquerque, New Mexico and Livermore, California, September 2012.
7 Somerday, B. P. and C. San Marchi, “Effects of hydrogen gas on steel vessels and pipelines,” SAND2006-1526P, Sandia National Laboratories, Albuquerque, New Mexico and Livermore, California, September 2006.
8 Murakami, Y., Metal fatigue: Effects of small defects of nonmettalic inclusions, 2nd Ed., Academic Press, Elsevier, London, UK, 2019.
9 American Society for Testing & Materials (ASTM) E1820-20b, “Standard test method for measurement of fracture toughness,” ASTM International, 2020.
10 International Organization for Standardization (ISO) 12135, “Metallic materials—Unified method of test for the determination of quasistatic fracture toughness,” 2021.
11 American Society for Testing & Materials (ASTM) E1681-03(20), “Standard test method for determining threshold stress intensity factor for environment-assisted cracking of metallic materials,” 2020.
12 American Petroleum Institute (API) API 579-1/ The American Society of Mechanical Engineers (ASME) FFS-1, “Fitness-for-service,” December 2021.
13 The American Society of Mechanical Engineers (ASME), “Boiler and pressure vessels code: Section VIII rules for construction of pressure vessels,” 2021.
14 American Society for Testing & Materials (ASTM) E647-15, “Standard test method for measurement of fatigue crack growth rates,” 2015.
15 International Organization for Standardization (ISO) 12108, “Metallic materials—Fatigue testing: Fatigue crack growth method,” 2018.
16 San Marchi, C., et al., “Technical basis for master curve for fatigue crack growth of ferritic steels in high-pressure gaseous H2 in ASME Section VIII-3 Code,” ASME Pressure Vessels & Piping Conference, PVP2019-93907, 2019.
17 Ronevich, J. A. and C. San Marchi, “Materials compatibility concerns for H2 blended into natural gas,” ASME Pressure Vessels & Piping Conference, PVP2021-62045, 2021.
18 Ronevich, J. A., et al., “Investigating the role of ferritic steel microstructure and strength in fracture resistance in high-pressure H2 gas,” ASME Pressure Vessels & Piping Conference, PVP2022-83915, 2022.
19 American Petroleum Institute (API) Recommended Practice 2Z, “Recommended practice for preproduction qualification for steel plates for offshore structures,” 4th Ed., 2005.
20 San Marchi, C., et al., “Fracture and fatigue of commercial grade API pipeline steels in gaseous H2,” ASME Pressure Vessels & Piping Division/K-PVP Conference, PVP2010-25825, 2010.
21 Ronevich, J., et al., “Oxygen impurity effects on H2 assisted fatigue and fracture of X100 pipeline steel,” ASME Pressure Vessels & Piping Conference, PVP2018-84163, 2018.
22 The American Society of Mechanical Engineers (ASME) Boiler and Pressure Vessels Code, “Code cases,” 2021.
23 European Standards, EN 13445, “Unfired pressure vessels—Part 3: Design,” 2021.
24 Miyamoto. T., et al., “Characteristics of fatigue life and fatigue crack growth of SCM435 steel in high-pressure hydrogen gas,” Nihon Kikai Gakkai Ronbunshu, A Hen/Transactions of the Japan Society of Mechanical Engineers, Part A 78(788), April 2012.
25 Macadre, A., et al., “Effects of hydrogen pressure and test frequency on fatigue crack growth properties of Ni-Cr-Mo steel candidate for a storage cylinder of a 70MPa hydrogen filling station,” Engineering Fracture Mechanics, Vol. 78, Iss. 18, December 2011.
26 Drexler, E. S., et al., “Fatigue testing of pipeline welds and heat-affected zones in pressurized hydrogen gas,” Journal of Research (NIST JRES), April 2019.
27 Stewart, A. T., “The influence of environment and stress ratio on fatigue crack growth at near threshold stress intensities in low-alloy steels,” Engineering Fracture Mechanics, 1980.
28 Wada, Y., “Effect of cycle frequency on fatigue crack propagation behavior for steels in hydrogen storage,” ASME 2013 Pressure Vessels & Piping Division Conference, PVP2013-97485, 2013.
29 Matsuo, T., et al., “Fatigue crack growth properties of quenched and tempered Cr-Mo steel in 0.7 MPa hydrogen gas,” 18th European Conference on Fracture: Fracture of Materials and Structures from Micro to Macro Scale, 2010.
30 Nibur, K. A., et al., “Fracture and fatigue tolerant steel pressure vessels for gaseous hydrogen,” ASME Pressure Vessels & Piping Division/K-PVP Conference, PVP2010-25827, 2010.
31 Somerday, B., et al., “Measurement of fatigue crack growth rates for Sa-372 Gr. J steel in 100 MPa hydrogen gas following Article KD-10,” ASME Pressure Vessels and Piping Conference, PVP2013-97455, 2013.
32 Ronevich, J., et al., “Measuring fatigue crack growth behavior of ferritic steels near threshold in high-pressure hydrogen gas,” ASME Pressure Vessels & Piping Conference, PVP2020-21263, 2020.
33 Nibur, K. A., et al., “Measurement and interpretation of threshold stress intensity factors for steels in high-pressure hydrogen gas,” SAND2010-4633, Sandia National Laboratories, Albuquerque, New Mexico and Livermore, California, 2010.
About the authors
MATTEO ORTOLANI is the Product Development Manager at Tenaris, and has worked as a metallurgist at the company since 2011. Dr. Ortolani has been involved with steel design and manufacturing process optimization of seamless tubular products for a variety of industrial applications, including power and process plants, structural and mechanical pipes, automotive products, tubular products and pressure vessels for high-pressure H2 storage and transportation. He is responsible for the engineering and development of products for industrial applications at Tenaris. Dr. Ortolani is a member of the ASME Boiler and Pressure Vessels Code Standards Committee on Materials (BPV II), and chairs the BPV II Sub-Group on Strength of Ferrous Alloys and the Working Group on Creep Strength-Enhanced Ferritic Steels.
PAOLO BORTOT is Product Manager at Tenaris, and has been involved with steel and pressure vessels design for H2 and embrittling gas transport and storage since 2007. Dr. Bortot is a member of several international working groups in the field of H2 material compatibility and high-pressure vessels design. He is presently a design engineer within the industrial technology department at Tenaris, and is a member of the ASME Boiler and Pressure Vessels Code B31.12, ISO TC58/WG7 and ISO TC197/WG15.